Math 117 Practice Final

Please work out each of the problems below.  Credit will be based on the steps towards the final answer.  Show your work.

Printable Key

Problem 1

Sketch the following.

A.  The point (3,4,1).

Solution

B.  The surface z  =  x² + y² 

Solution

C.  Five level curves to the surface z  =  x - y² 

Solution

Problem 2

Find f_xz for

        f(x,y,z)  =  x²z + y² - y cos(xz)

Solution

Problem 3

A swimwear store sells both men's and women's swimsuits and has determined that the profit, P,  it can make in stocking x men's suits and y women's suits is 

        P(x,y)  =  2x² + y² + xy - 900x - 1100y  + 400,000

Determine the number of men's and the number of women's suits that should be stocked in order to maximize profit.  Then determine the maximum profit.

Solution

Problem 4

A medical researcher is studying the effect that exercise has on a women's percent body fat.  The table below shows the results of the corresponding study done.  Determine the least squares regression line for this data and use it to estimate the mean percent body fat for a women that exercises 3 hours per week.

Solution

Problem 5

Switch the order of integration to evaluate the following

Solution

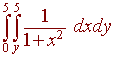
Problem 6

Consider the following game.  Pick a card.  If you select a face card (Jack, Queen, or King) you win $3.  Otherwise, you lose $1.  Find the expected value and comment on how this number shows whether playing this game many times is a good idea.

Solution

Problem 7

Consider the following probability density function

        f(x)  =  x + 0.5        0  <  x  <  1

A.  Find P(0.5 < x < 1).

Solution

B.  Find the standard deviation.

Solution

C.  Find the median.

Solution

Problem 8

A pair of baby rabbits is introduced to an island.  Assume that rabbits take two months to reproduce when they have 2 pairs of new baby rabbits. Each month thereafter, the adult rabbits produce two additional pairs of rabbits. Assume that the rabbits never die.

A.  Write down the number of pairs of rabbits on the island during the first 6 months.

Solution

B.  Find constants u and v such that

        a_n  =  u a_n-1 + v a_n-2 

Where a_n represents the number of rabbits after the n^th month.

Solution

Problem 9

A very long river that runs along a vast area of farming communities has 3000 trout in its first mile.  Because of pollutants, each mile contains only 80% of the fish that the previous mile had.  so that the second mile has 2400 trout and the third has 1820 trout.  Considering the river as running an infinite distance, how many trout live in the river?

Solution

Problem 10

Determine whether the following series converge or diverge.  Be sure to cite the test that you are using.

A. 

Solution

B. 

Solution

Problem 11

Find a power series representation for 

using the fact that

then find the radius of convergence for your solution

Solution

Problem 12

Find the third degree Taylor polynomial centered at x  =  0 for

        f(x)  =  x e^x 

Solution

Problem 13

Use Newton's method  with initial guess of 1 to estimate 

within three decimal places of accuracy by finding the solution to 

        x²  -  2  =  0

Solution

Problem 14

Solve the following differential equation

                    1
        y ' -             y  =  2x² + 1      
                    x

Solution

Problem 15

The rate of growth of a bacteria is equal to the quotient of the number of bacteria present and one more than the number of hour that the bacteria has been growing.  If initially there were 5 grams of bacteria, how many grams of bacteria will there be in 8 hours?

Solution