Math 152B Practice Exam II

Please do all of the following problems.  All work and all answers must be done on your own paper.  Credit earned will be based on the steps that you show that lead to the final solution.  Good Luck!

 

Problem 1   Find the root:

           

Solution

        -2,  since (-2)3 = -8

 

 

Problem 2   Simplify the expression.  Leave your answer in exponential form with only positive exponents.

        

 Solution

       

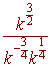
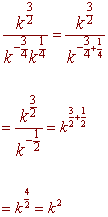
Problem 3  Multiply and Simplify  

       

Solution

       

 

Problem 4   Simplify the expression.  Assume all variables represent positive real number.

          

 Solution

       

 

 

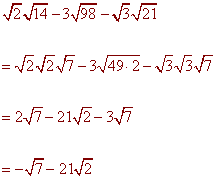
Problem 5    Multiply and Simplify

        

   Solution

               

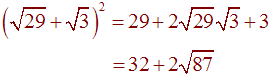
Problem 6  Rationalize the denominator

              5
                       
          4 -

 Solution

                     5    (4 + )
                                                
                 ( 4 - )(4 + )

 

                      20 + 5
             =                          
                        16  -  6

 

                      20 + 5
             =                          
                            10

 

Problem 7   Simplify        

        

 Solution

       

 

 

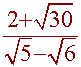
Problem 8 

 Use the method of elimination to solve the system.  Then graph the system to check your solution.

  1.  -3x + 2y = 6
      9x - 6y  =  36

    Solution

    We multiply the first equation by 3 and add

          -9x + 6y = 18
            9x - 6y  =  36

            0  =  54

    This is a contradiction, so there is no solution.
          
    To graph it we have make T-Tables as shown below

      
    x y
    0 3
    -2 0

     
    x y
    0 -6
    4 0

    The graphs are shown below.  Notice that they are parallel.

           

     


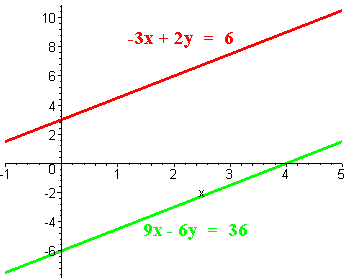
  2. 5x + 3y  =  15
    3x - 2y  =   28
     

    Solution

    Multiply the first equation by 2 and the second by 3 and add

                10x + 6y  =  30
                 9x   - 6y  =  84

                19x  =  114     
                    x  =  114/19  =  6

    Now substitute this value into the second equation (the first will work also) to get

                3(6) - 2y  =  28

                18 - 2y  =  28

                -2y  =  28 - 18  =  10

                y  =  10/-2  =  -5

    The solution is (6, -5).

    To graph it we have make T-Tables as shown below

      
    x y
    0 5
    3 0

     
    x y
    0 -14
    28/3   0

    The graphs are shown below.  Notice that the lines intersect at (6,-5).

           

     

 

  Problem 9

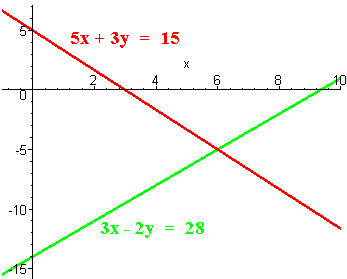
Use the method of substitution to solve the system.  Then graph the system to check your solution.

  1. 7x + y  =  3
    4x + 3y  =  -8
     

    Solution

    We solve the first equation for y:

            y  =  3 - 7x

    Now plug this into the second equation:

            4x + 3(3 - 7x)  =  -8

            4x + 9 - 21x  =  -8

            9 - 17x  =  -8

            -17x  =  -17

            x  =  1

    Now plug in x = 1 in the equation

            y  =  3 - 7(1)  =  -4

    Hence the solution is (1,-4).

    To graph it we have make T-Tables as shown below

     
    x y
    0 3
    3/7 0

     
    x y
    0 -8/3
    -2   0

    The graphs are shown below.  Notice that the lines intersect at (1,4).

           

     

     

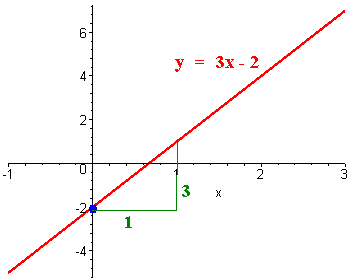
  2. y  =  3x - 2
    6x - 2y  =  4
     

    Solution

    We can substitute the first equation into the second:

            6x - 2(3x - 2)  =  4

            6x - 6x + 4  =  4

            4  =  4

    This is an identity, hence there are an infinite number of solutions (all points lying on the line y  =  3x - 2).  Notice the the y-intercept is -2 and the slope is 3.  We can draw the point (0,-2) and rise 3 and run 1 from this point to graph the line.

           

     

 

  Problem 10   Solve       

         

 Solution

        Square both sides:

                a2 + 24  =  (a - 2)2

                a2 + 24  =  a2 - 4a + 4

                24  =  -4a + 4

                4a  =  -20

                a  =  -5

            Notice that when we plug x  =  -5 into the right hand side of the original equation, we get -7, while the left hand side produces 1.  Hence there is no solution.

 

Problem 11  Solve each inequality.

A.  | 2x - 4 | + 3 > 7

Solution

First subtract 3 from both sides

        |2x - 4| > 4

Now turn this into an "or" statement

        2x - 4 = 4 or 2x - 4 = -4

        2x = 8  or  2x = 0

        x = 4  or  x = 0

Now test the point x = 1 to see if we yield a true statement

        |2(1) - 4| + 3  =  |-2| + 3  =  2 + 3  = 5

is not greater than 7.  Hence the solution is the outside two intervals:

        (- , 0) U (4, )

 

B.  | 3x - 8 | + 5  < 4

Solution

First subtract 5 from both sides of the inequality

        |3x - 8| < -1

Now notice that the absolute value can never be negative, in particular it is never less than -1.  Hence there is no solution.

 

C.  3| x - 5| <  9

SolutionFirst divide both sides by 3 to get 

       |x - 5|  <  3

Now turn this into an "or" equation.

        x - 5 = 3  or  x - 5 = -3

        x = 8  or  x = 2

Now test a between point such as 5:

        |(5) - 5| = 0 which is less than 3, hence the between interval is the solution.  The solution is

        (2,8)

 

Problem 12  Solve the equation

        x - 3       x - 6
                 -               =  0
       x + 1       x + 5

Solution

        Multiple all three terms by the common denominator (x + 1)(x + 5)

        (x - 3)(x + 1)(x + 5)            (x - 6)(x + 1)(x + 5)
                                           -                                    =  0
                 x + 1                                 x + 5

        (x - 3)(x + 5)  -  (x - 6)(x + 1)  =  0

        [x2 - 3x + 5x - 15]  -  [x2 - 6x + x - 6]  =  0

        [x2 + 2x - 15]  -  [x2 - 5x  - 6]  =  0

        x2 + 2x - 15  -  x2 + 5x  + 6  =  0

        7x - 9  =  0

        7x  =  9

        x  =  9/7

 

Problem 13  Solve the following for m

                             r
        S  =  1  +            
                         m

Solution

first subtract 1 from both sides

                           r
        S - 1  =             
                       m

Now multiply both sides by m

        m(S - 1)  =  r

Now divide both sides by S - 1

                          r
        m  =                    
                    S - 1

       

Problem 14  Steve can paint his house in 10 hours working by himself.  Working together, Anne and Steve can paint the house in just 6 hours.  How long would it take Anne to paint the house by herself?

Solution

Since Steve can paint the house in 10 hours, he paints the house at the rate of 1/10 houses per hour.  If Anne can paint the house in x hours, she paints at a rate of 1/x houses per hour.  The sum of the individual rates equal the total rate (1/6 houses per hour).  

              1            1         1 
                  +          =             
          10           x          6  

Now multiply by the common denominator 30x

              1 (30x)           1(30x)        1 (30x)
                          +                 =                  
             10                   x                6  

        3x + 30  =  5x

        2x  =  30

        x  =  15

It would take Anne 15 hours to paint the house by himself.

 

Problem 15  Derek bicycled 36 miles to get to Echo Summit and back and Nick bicycled 60 miles to get to Carson Pass and back.  Nick rode 3 miles per hour faster than Derek, and his trip took an hour longer than Derek's.  What is the fastest speed that Derek could have been traveling?  (You must set up the equations, that is, no guessing).

Solution

We construct the following Distance-Rate-Time table

  Distance Rate Time
Derek 36 r t
Nick 60 r + 3 t + 1

This gives us the two equations

        36  =  rt        60  =  (r + 3)(t + 1)

The first equation gives us

        t  =  36/r

so that

        60  =  (r + 3)(36/r + 1)

Multiplying both sides by r gives

        60r  =  (r + 3)(36 + r)  =  r2 + 39r + 108

or

        r2 - 21r  + 108  =  0

        (r - 9)(r - 12)  =  0

So that

        r  =  9     or      r  =  12

The fastest that Derek could have been traveling is 12 miles per hour.

 

Problem 16  Simplify

                          x
           3 -                          
                        x + 1
                                          
             x            1
                    +                      
             6            4

Solution

We first find the least common denominator.  The denominators are  x + 1, 6, and 4.  The LCD is

        LCD  =  12(x + 1)

Now multiply the two terms in the numerator and the two in the denominator by the LCD

                                      x        12(x + 1)
           3(12)(x + 1) -                                
                                   x + 1           1   
                                                                              
             x       12(x + 1)             1      12(x + 1)
                                         +                                    
             6             1                  4             1

Now cancel to get

                       3(12)(x + 1)  - 12x
            =                                             
                       2x(x + 1) + 3(x + 1)

Distribute to get

                       36x + 36  - 12x
            =                                     
                     2x2 + 2x + 3x + 3

Combine like terms

                      24x + 36
            =                           
                    2x2 + 5x + 3

Factor the numerator and the denominator

                        12(2x + 3)
            =                               
                     (2x + 3)(x + 1)

Now cancel the x + 1 to get

                      12
            =                 
                    x + 1

 

Back to the Factoring and Rational Expressions Page

Back to the Basic Algebra Part II Page

Back to the Math Department Home Page

Questions, Comments and Suggestions  Email:  greenl@ltcc.edu